Rules Of Mathematics Algebra Pdf

Basic of Algebra

Algebra is the field of mathematics which deals with representation of a situation using mathematical symbols, variables and arithmetic operations like addition, subtraction, multiplication and division leading to the formation of relevant mathematical expressions. In this lesson we will go through all the rules of algebra, operations and formulas.

1.	Algebra Basics
2.	Algebra Rules
3.	Algebraic Operations
4.	Algebraic Formulas
5.	Solved Examples on Basics of Algebra
6.	Practice Questions on Basics of Algebra
7.	FAQs on Basics of Algebra

Algebra Basics

We need to know the basic terminology which relates to algebra in order to understand its basics. An expression consisting of 4 main parts, variables, operators, exponents, coefficients and constants along with an equal to symbol is known as an algebraic equation. Let us take an equation, ax² + bx + c = d. In algebra, the term with highest exponent is written in the starting and further the terms are written with reducing powers.

Algebraic Equation Parts

In the above imageax ² + bx + c = d, there are 4 terms. An algebraic equation may have different terms which are like or unlike. Like terms in an equation are the ones which constitute same variables and exponents. On the other hand, unlike terms in an equation constitute different variables and exponents.

Algebra Rules

There are five basic rules of algebra. They are:

Commutative Rule of Addition
Commutative Rule of Multiplication
Associative Rule of Addition
Associative Rule of Multiplication
Distributive Rule of Multiplication

Commutative Rule of Addition

In algebra, the commutative rule of addition states that when two terms are added, the order of addition does not matter. The equation for the same is written as, (a + b) = (b + a). For example, (x³ + 2x) = (2x + x³)

Commutative Rule of Multiplication

The commutative rule of multiplication states that when two terms are multiplied, the order of multiplication does not matter. The equation for the same is written as, (a × b) = (b × a). For example, (x⁴ - 2x) × 3x = 3x × (x⁴ - 2x).
LHS = (x⁴ - 2x) × 3x = (3x⁵ - 6x²)
RHS = 3x × (x⁴ - 2x) = (3x⁵ - 6x²)
Here, LHS = RHS, this proves that their values are equal.

Associative Rule of Addition

In algebra, the associative rule of addition states that when three or more terms are added, the order of addition does not matter. The equation for the same is written as, a + (b + c) = (a + b) + c. For example, x⁵ + (3x² + 2) = (x⁵ + 3x²) + 2

Associative Rule of Multiplication

Similarly, the associative rule of multiplication states that when three or more terms are multiplied, the order of multiplication does not matter. The equation for the same is written as, a × (b × c) = (a × b) × c. For example, x³ × (2x⁴ × x) = (x³ × 2x⁴) × x.

Distributive Rule of Multiplication

The distributive rule of multiplication states that when we multiply a number to addition of two numbers, it results in the output which is same as the sum of their products with the number individually. This is distribution of multiplication over addition. The equation for the same is written as, a × (b + c) = (a × b) + (a × c). For example, x² × (2x + 1) = (x² × 2x) + (x²× 1).

Algebraic Operations

The four basic algebraic operations are:

Addition
Subtraction
Multiplication
Division

In each of the algebraic operations performed, we always categorize the terms in our algebraic equations as like and unlike terms.

Addition

When two or more terms in an algebraic equation are separated by a plus sign "+", the algebraic operation is addition. We always add the like terms and unlike terms separately as they are treated as two different quantities. Mathematically two different quantities cannot be added together.

Example of like terms addition: 5b + 3b = 8b
Example of unlike terms addition: 25x + 35y

As we can see in the examples, the like terms when added give the same term while the unlike terms cannot be added any further.

Subtraction

When two or more terms in any algebraic equation are separated by a minus sign "-", the algebraic operation is subtraction. Just as in case of addition, the terms are differentiated as like or unlike terms and then subtracted further.

Example of like terms subtraction: 3x² - x² = 2x²
Example of unlike terms subtraction: 6bc - 9ab

Multiplication

When two or more terms in an algebraic equation are separated by a multiplication sign "×", the algebraic operation performed is multiplication. While multiplying the like terms or unlike terms we use Laws of Exponents.

Example of like terms multiplication: 16f × 4f = 64f²
Example of unlike terms multiplication: x × y³ = xy³

Division

When two or more terms in any algebraic equation are separated by a division sign "/", the algebraic operation performed is division. While dividing the like terms, the similar terms can be simplified while for the case of unlike terms, the terms cannot be simplified any further easily.

Example of like terms division: 8b/2b = 4
Examples of unlike terms division: x²/2y²

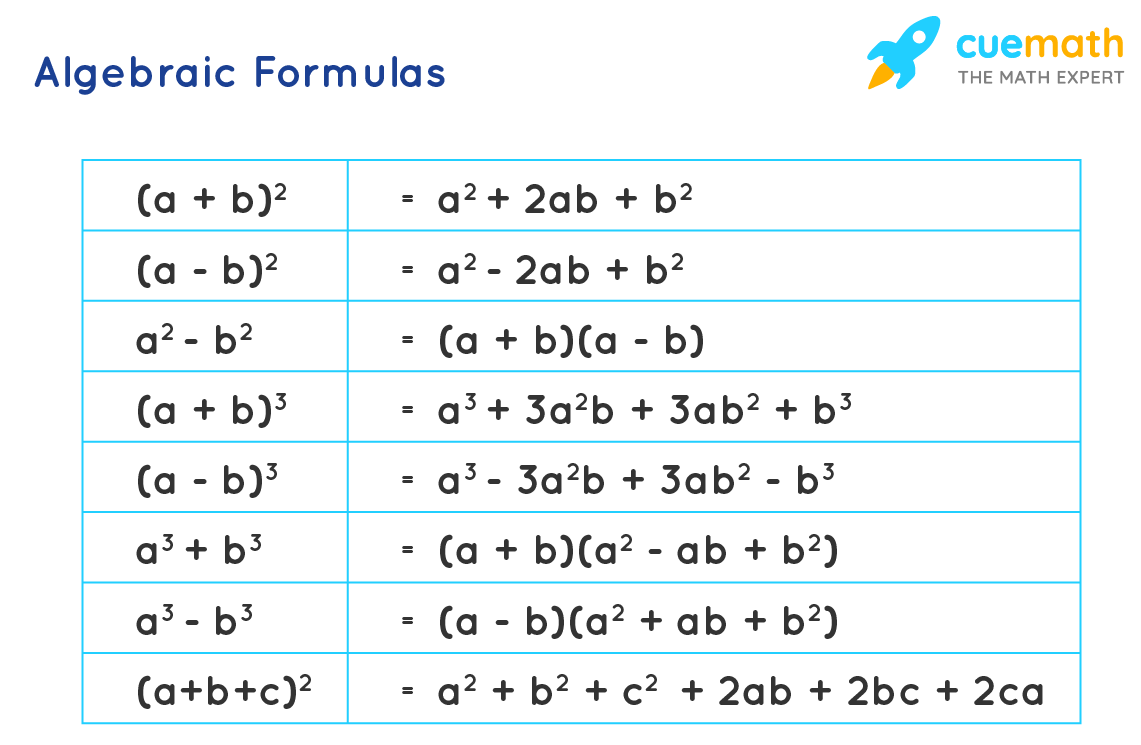
Algebraic Formulas

The algebraic formulas that are used more often and must be kept in knowledge are:

Formulas in Algebra

Topics Related to Basics of Algebra

Algebra
Variables, Constants and Expressions
Exponents
Basic Algebra Formula
Addition of Algebraic Expressions
Subtraction of Algebraic Expressions
Division of Algebraic Expressions

Example 1: Simplify the given algebraic equation.

x³ - 4x² + 3x + 5x² - 8x + 3x³ - 5 = 0

Solution: We can simplify the given equation by grouping like terms and unlike terms.
x³ - 4x² + 3x + 5x² - 8x + 3x³ - 5 = 0
\(\implies\) (x³ + 3x³) + (5x² - 4x²) + (3x - 8x) - 5 = 0
\(\implies\) 4x³ + x²- 5x - 5 = 0
\(\therefore\) The given algebraic equation can be simplified as,4x³ + x²- 5x - 5 = 0
Example 2: Prove that x³y²and x²y³ follow commutative rule of multiplication.

Solution: The commutative rule of multiplication follows (a × b) = (b × a)
Let a = x³y² and b = x²y³
(a × b) = (x³y²× x²y³) = x⁵y⁵
(b × a) = (x²y³× x³y²) = x⁵y⁵
As (a × b) = (b × a) = x⁵y⁵
\(\therefore\) The given terms follow commutative rule of multiplication.

go to slidego to slide

Have your child solve real-life challenges using math

Have your child apply concepts learned in school in the real world with the help of our experts.

Book a Free Trial Class

FAQs on Basics of Algebra

What are the Basic Rules in Algebra?

The basic rules in algebra are:

Commutative Rule of Addition
Commutative Rule of Multiplication
Associative Rule of Addition
Associative Rule of Multiplication
Distributive Rule of Multiplication

What is the Golden Rule in Algebra?

The golden rule in algebra is to keep both sides of the equation balanced, i.e; whatever operation is being used on one side of equation, the same will be used on the other side too.

What are the Four Algebraic Operations?

Addition
Subtraction
Multiplication
Division

How Do You Add and Subtract Like Terms?

When like terms are added or subtracted, the coefficients are added or subtracted and written before the like terms.

Can We Add or Subtract Two Unlike Terms?

No, we cannot add or subtract two unlike terms.